| Tunes | Improvisations | Bird Song | New Fractal Tunes | Recordings | Test my Midi player |
Intro, The major chord as a triangle, Pure or just intonation, The minor chord as a downward pointing triangle, Lattice keyboards, Into the third dimensison - musical sculpture, The cubic lattice of chords, Into the fourth space dimension and higher
Visit the Test my Midi player page before you listen to these clips - to find out if you can hear them tuned as intended.
When you click on one of the pictures on this page, you may hear a clip played in the background, or it may get played in a separate midi player, or it may open a new web page each time, depending on the setup for your browser and midi player. In particular, Quicktime normally plays them in a new page.
This page work best if you have the type of set up where the clip plays in the background or a separate midi player.
If you have the type of setup where the clip gets played in a new window each time, be sure to use the new window version of this page
There's a java applet of the hexany too: - Java hexany.
This page explores links between chords in music and geometrical shapes. This idea goes back to at least the nineteenth century when hexagonal or square layouts for musical keyboards were explored. These are designs for two dimensional arrays of keys rather than the one dimensional single row of keys (black or white) we are used to in a normal music keyboard.
You can do the same in three dimensions too, and it is used in this way in modern tunings such as the CPS sets of Erv Wilson. The idea of a two dimensional keyboard gets generalised into a kind of musical interactive sculpture in 3D - you can click on the vertices to hear notes and the faces then correspond to triads which are interconnected in the same fashion as the faces of the geometrical shape. These sculptures give one highly symmetrical arrangements of triads, often with no tonal centre, because of the symmetry of the shape, so the shape you see helps one to appreciate the way the chords are related to each other. These tunings are also used in musical compositions - particularly by Kraig Grady, and the result is music with a weightless floating quality.
So that gives you an idea of the context; lets get down to the nitty gritty.
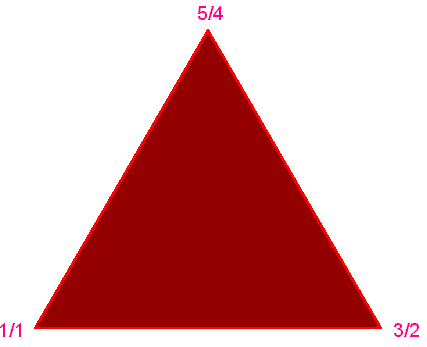
A triad in music is made of three notes. A triangular face in geometry has three vertices. So how about placing a note at each vertex of a face like this - I'll explain the numbers in a moment:

Click on any of the numbers to hear a note. Click on an edge to hear a two note chord of two of the notes playing together. Click in the centre and you will hear all the three notes playing together in a harmonious chord.
Now to explain the numbers. We hear notes in terms of ratios, so that for instance if one note is double the frequency of another it will always sound an octave apart. So for instance, the jump from 110 to 220 Hertz is an octave, and so is the interval from 440 to 880 Hertz. Therefore, it makes good sense to notate the octave as 2 for doubling. Actually the convention is to write it always as a ratio even for whole numbers, like this: 2/1. One writes 1/1 for the unison.
Some ratios sound particularly consonant. For instance the ratio 3/2 from say 440 to 660 Hertz is a particularly consonant one - it is the interval from A to E in music - a fifth (i.e. an interval of five notes A B C D E).. Another nice one is 5/4 which is the interval from A to C#, 440 to 550 Hertz, a major third (three notes in the scale of A major: A B C#). Then the interval from C# to E from 550 to 660 Hertz is 6/5 which is also consonant. Those intervals together make a major chord.
Strictly speaking, we should call the chord we have just made the pure or just major chord..
The modern equal tempered piano tuning uses an approximation to the pure major third for the interval from A to E, It is about 15 cents sharp compared with the pure and most consonant version of this interval.
How the interval sounds depends on the instrument timbre -this is for harmonic timbres, which include voice, strings, and in fact most of the melodic instruments of an orchestra. It is especially noticable for melodic timbres rich in overtones such as voice, strings, and harpsichord. However, for bells and gongs, the component frequencies or "partials" of the timhre are arranged in another kind of a way - they are known as inharmonic timbres, and the way they work in chords is a little different.
The equal tempered major chord sounds fine to most of us nowadays, but sometimes one notices that it beats and sounds rather rough. This is especially noticeable in some types of music such as harpsichord music. It was used for the tuning of lutes quite early on. However, the tuning only caught on for keyboards in the late nineteenth and twentieth centuries - partly for practical reasons certainly, but possibly because of the way it sounds too - the beating and roughness (or one could say brightness) of the chords is more noticeable on a harpsichord than on a lute. It may be something of an aquired taste. If one listens to it after maybe a few weeks immersion in just intonation, twelve equal can sometimes have a rather exotic kind of a "Gamelan" like quality to it.
Then in Bach's time, well temperaments became popular, which allow you to modulate to any key. At that time, the C major key might still have pure major thirds. Later, as time went on, composers began to modulate more and more freely, and the tunigns used developed accordingly. For instance, a typical tuning of a keyboard of Mozart's time is at most 8 cents away from equal temperament - while a typical tuning of Bach's time goes up to 15 cents away from equal temperament
The major third is flatter than the equal tempered one and the minor third is sharper. The fifth is pretty close to the equal tempered one, within two cents (two percent of a semitone)
Singers in choirs who sing a capella generally fall into pure ratios when they sing long sustained chords - measurements have been made to confirm this, and gradually they move closer to the pure ratios as the chord sustains longer.
If you are interested to follow this up a bit further and find out why the notes of the pure major chord sound good together, see Harmonics and just temperament. Here, just intonation is a techinical term, and means tuning according to ratios (often fairly small numbered ratios) - it is the usual word for it in fact. If you want to find out about the notation and the maths, see cents and ratios. Also, see the Newbie Notes on my tunes page.
Okay, let's review what we've discovered so far, and go on to find out about the minor chord.
This is the pure major chord:
As ratios from the unison: 1/1 5/4 3/2 As ratios from the previous note: 5/4 6/5
The pure minor chord is the inversion of the major chord:
As ratios from the unison: 2/3 4/5 1/1 As ratios from the previous note: 6/5 5/4
Here we have turned all the ratios on their heads: 3/2 changes to 2/3, 5/4 to 4/5 and so on.
The result is a minor third followed by a major third such as say, A to C and C to E- the inverse of the major chord which consists of a major third followed by a minor one, such as say C to E then E to G..
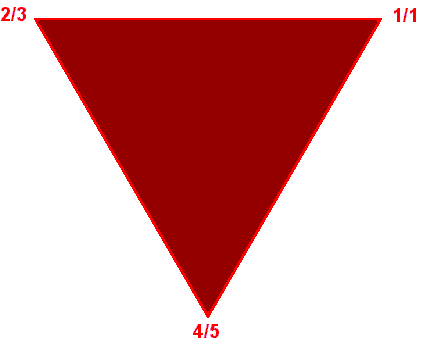
Again, click in the middle of any edge to hear the diad (two note chord) and click in the middle to hear the triad.
You may have heard of the "Circle of fifths" in music. The idea is that you can keep going up by fifths, or alternatively, down by fifths, like this
Since we hear notes an octave apart as "the same note", we can multiply or divide by 2 as much as we like to get them all into the same octave, like this:
The chord progressions in modern "common practice" twelve tone music are based on this. For instance one will often use the sequence: A major chord, E major chord, A major chord (or I, V, I if you use that notation).
Well, what you've done there is to start with a triad based on the 1/1 as A major, then moved up to another one based on the fifth of the previous chord, i.e.the 3/2, so you've gone up one chord in the circle of fifths. Then you go back down again.
Or one may go 1/1 3/2 1/1 4/3 1/1 (I ,V, I, IV, I),
If we play that as chords, maybe you will recognise it as a
commonly used cadence:
1/1 chord
3/2 chord
1/1 chord
4/3 chord
1/1 chord
various other progressions are possible going up / down this cycle of chords.
Now, there's a circle of major thirds as well, like the circle of fifths:
When we put both of those together, we get the two dimensional chord lattice:
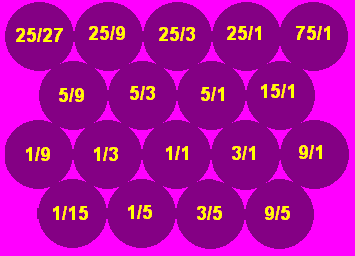
Here, when you move to the right you multiply the numbers by 3 When you move diagonally up to the right you multipy by 5. All the numbers get reduced into the same octave as the 1/1, but to make the diagram clearer, the octave multiples of two are left out. For example, starting from the 1/1, the notes actually contine to 5/4 and 25/16 diagonally up to the right, also to 3/2 and 9/8 to the right.
So every horizontal row of numbers makes a circle of fifths, and every column diagonally up to the right is a circle of major thirds.
Anyway the result is that every triangle of notes plays a major or minor triad. Upward pointing triangles play the major chords and downward pointing ones play minor chords. So for instance, 1/1 5/1 3/1 plays the major chord - actually 1/1 5/4 3/2 when reduced into the octave. Similarly, 1/1 3/5 3/1 plays the minor chord 1/1 6/5 3/2.
Then as you see, they all dovetail together so that each chord shares two notes with its neighbours in all directions.
To hear it, click on the numbers to hear the notes, and to hear the major and minor triads, click on the gaps between the circles where three of the numbers meet. Then try going from chords to adjacent chords horizontally or diagonally and listen to the chord progressions. For instance, if you go to the right and then back again, that's a I V I chord progression. Go to the right twice, left three times and right once to get back to where you started and you will play a I V II V I IV I chord progression, which is a frequently used progression in music for extended cadences.
Keyboards have actually been built following this pattern - and the neat thing about it is that you only need to learn one fingering to play in any scale you please. This picture uses fifths and major thirds, but other arrangements are also possible, such as say, minor thirds and seconds, or whatever. Each method requires its own fingering system, but then once you've learnt it, you can play in any key you like in the same way.
Okay, that covers all that one needs to know to understand the connection between common practice music and the pure just intonation triads. Notice though that our musical palette consists entirely of numbers which are multiples of 3 or 5.
One may want to go beyond that to explore other kinds of tonalities involving the numbers 7, or even 11 and 13. The number 7 is a great favourite with many mirotonal composers. Actually, musicians who don't know much about all this may slip into it naturally too as it can sound nice. If you play a minor third to sound as pure as possible, you may play it a bit sharper than the twelve equal one - if so you are probably playing a pure minor third at 6/5 - because the pure minor third is sharper than the equal tempered one.
However, you may find that you play your minor third very flat instead compared with the equal tempered one - this is quite commonly done - then you get a very attractive dark sombre kind of a minor third. 'That's the septimal minor third, 7/6. Rather kind of leisurely too, makes ones playing seem very relaxed
Another place where 7 comes in is in the dominant seventh chord. The usual just intionation tunings of the dominant seventh for common practice music are 1/1 5/4 3/2 16/9, or 1/1 5/4 3/2 9/5. There are two versions because you have two sizes of tone in the lattice - 10/9 and 9/8 - i.e. the interval between the 1 and the 9, or the 1 and the 5/9, when reduced into the octave.
However, probably the most consonant possible dominant seventh is the one with a 7/4: 1/1 5/4 3/2 7/4.
Here is a clip of the three dominant sevenths to compare:
and if you want to go to a resolution after all those dominant sevenths, here is the major chord major chord on the 4/3.
Don't expect a striking difference, but in a musical cadence, the 7/4 dominant seventh is more mellow and relax, while the 16/9 one is a bit more decisive, and the 9/5 one is perhaps somewhat between the two.
The corresponding circle now is by 7s instead of 5s or 3s:
and instead of a two dimensional lattice made up of triangles, you get a three dimensional lattice made up of tetrahedra and octahedra.
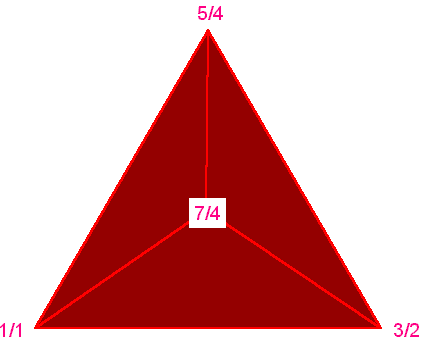
Here is a tetrahedron from the lattice, with the major triad as before, and the 7/4 added in. Again click on the numbers to hear the notes. Click in the middle of the faces to hear the triads. Click outside the shape to the left to hear the major triad (because it's face is underneath in this picture, so you can't see it). and click anywhere outside it to the right to hear the complete four note chord (tetrad).
Here the circle of 3/2s runs from left to right, the 5/4s go diagonally up to the right and the 7/4s are towards you and to the right. This is part of a three dimensional lattice - in fact it's the same arrangement that is used by green-grocers for stacking oranges and apples. If you look at the spaces in a stack of oranges, you find that sometimes there are four neighbouring oranges, and sometimes six, so the lattice is built out of both tetrahedral and octahedral clusters of spheres.
The octahedron works the same kind of a way. Here is a picture:
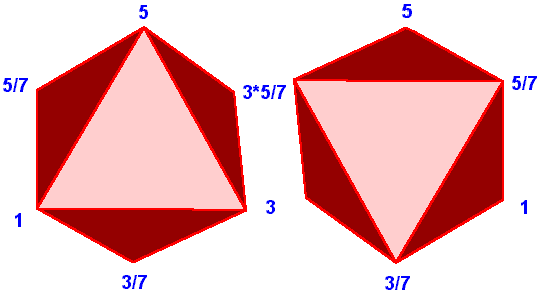
Click on the red spheres to hear the triads. Each triad shares a diad of two notes with all its three closest neighbours.
The picture shows the octahedron from both sides. The light coloured face on the left image is the major chord, the bottom one is the minor chord, and the shaded ones are various septimal minor and major chords. Wonderfully dark sounding mysterious chords :-).
Chords of this type are called otonal or utonal if they are based on the overtone series 1/1 2/1 3/1 4/1 5/1 ... or the undertone series 1/1 1/2 1/3 1/4 1/5 ... respectively. So utonal chords are the ones that when expressed in their simplest form, and ignoring the octaves, have the numbers on the top like this: 1/1 5/1 3/1 (major chord). Utonal ones have them on the bottom like this: 1/1 1/5 1/3 (minor chord).
Notice that 1/1 5/1 3/1 is the same chord as 15/15 15/3 15/5, i.e 1/15, 1/3, 1/5. Following that same pattern, all otonal chords are also utonal and vice versa. The chord is otonal if it is otonal when expressed in the simpler of the two forms, i.e. with the numbers as small as they can get.
You can call it septimal if it uses the number 7 in any of the ratios.
All the septimal (shaded) chords in the left-most image are utonal chords. The chord at the bottom, 1 3 3/7 is 1/3 1/1 1/7 (after dividing through by 3 - if you divide all the numbers in a chord by the same amount you get the same chord just transposd in pitch - so the same flavour e.g. otonal or utonal). The other two septimal utonal chords are 1/1 1/5 1/7 and 1/3 1/5 1/7 (after dividing by 15).
The septimal chords on the right are all otonal, for instance multiplying 1 3/7 5/7 by 7 you get 1 3 7, and the others are 3 5 7 and 1 5 7 after multiplying by suitable numbers.
The septimal minor chord (also known as the subminor third) which you may have heard of, and got mentioned earlier in this page, is 1/1 7/6 3/2, i.e. 1/1 7/3 3/1 up to octave equivalence In otonal form it is. 3 7 9, and in utonal form it is 1/21 1/9 1/7, so it is actually an otonal chord. It's not in this particular shape - none of the otonal chords have a 9/7 diad in them which you would need for the standard septimal minor chord..
Microtonal composers and theorists call this shape the hexany, because it has six points
It's usually presented as 1*3, 1*5, 1*7, 3*5, 3*7, 5*7 - using all multiples of pairs of numbers from 1, 3, 5 and 7. The version shown here is 1, 3, 5, 3/7, 5/7, 3*5/7 and you get from this one to the hexany in its standard presentation by transposing all the notes up in pitch by 7 in the lattice (up to octave equivalence of course), i.e. move up one layer in the lattice in the 7/1 direction.
It gets called the 1 3 5 7 hexany because it uses pairs of numbers chosen from 1, 3, 5 and 7
If you want to find the hexany with the usual septimal minor chord in it, you need the 1 3 7 9 hexany. I'll do a picture of one of those too, with musical clips as before, and add it in here presently. (P.s. - sorry haven't got around to doing it yet).
The green grocer's stacks of oranges are actually often stacked with a square arrangement of oranges in the bottom layer, because then you get nicely formed pyramidal stacks. It's the same arrangement of octahedra and tetrahedra, just tilted so that the layers of octahedra are horizontal.
If we show the 3D lattice this way, we can get a better idea about how the octahedra and tetrahedra fit together. Here is the 3D lattice again, tilted so that you see one of the layers of octahedra/
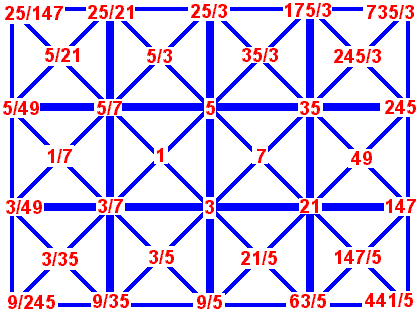
Click on the numbers to hear the notes. Click in the middle of any of the edges within the picture to hear the tetrads. The edges around the border of the picture don't sound.
The picture shows a sheet of the octahedra from above - the 1/7, 1, 7 etc are at the tops of the octahedra.
Then to see how the tetrahedra fit in, imagine a line drawn from say the 1 to the 7. Then the 1, 3, 5 and 7 make a tetrahedron. The tetrad you hear when you click on the middle of the edge from 5 to 3 is the 1 3 5 7 tetrad.
The centres of the tetrahedra lie on a cubic lattice. Gene Ward Smith calls this the cubic lattice of chords.
Then it can be nice to have both types of chord in the same lattice, - but then you need four directions. 1 3 5 7 9 - so you go up into the fourth space dimension ! :-). The fourth and higher dimensions are also needed if you want to look at chords involving the numbers 11, or 13 etc. I will say a bit more about those here presently. Meanwhile, the old page about all this is the one called Exploring chords in the Wilson CPS sets
The midi clips in this page were made using Fractal Tune Smithy The way it works is that you use the notation for the notes you want to hear as the file name. E.g. "3/7 5/7 3*5/7mid". FTS converts the file names to 3o7_5o7_3s5o7.mid to make them suitable for use in a file name or url. Here s means * and o means /. Underlines stand in for spaces, You could also use this notation yourself when entering the names of the clips in the first place.
Then once you are finished making your page, you get FTS to read through the page, and it automatically makes all your clips for you - all these clips were made in FTS with a single click of a button. More details are explained in FTS | Help | FAQ | Music Making | How do I use the feature to make midi clips for all the file names in a web page?